How to Calculate Inertia for Standard Shapes
Inertia, specifically rotational inertia or moment of inertia, not only depends on how much mass an object has, but also on how that mass is distributed relative to the axis of rotation.
Different shapes have different geometries, which causes their mass to be positioned at varying distances from the axis. The farther the mass is from the axis, the greater the resistance to rotational motion.
For example:
-
A solid cylinder has more mass concentrated near the axis → lower moment of inertia.
-
A hollow cylinder has mass farther from the axis → higher moment of inertia.
-
A thin rod rotating about its centre has less resistance than the same rod rotating about one end.
This is why each shape has its own unique formula for moment of inertia—reflecting both its mass and geometry.
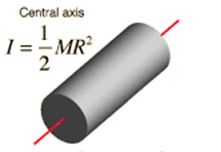
Solid Cylinder
A solid cylinder is a common shape in engineering, found in components like rollers, shafts, and flywheels. When it rotates about its central (longitudinal) axis, its moment of inertia is given by:
I = ½ MR2
Example
A solid cylinder has:
- Mass M = 8 kg
- Radius R = 0.3 m
I = ½ MR2
I = ½ × 8 × (0.3)2
I = 0.5 × 8 × 0.09 = 0.36 kg⋅m2
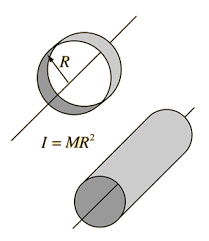
Hollow Cylinder
A hollow cylinder (also called a cylindrical shell) is a tube-like structure where all the mass is concentrated at a fixed distance from the central axis. When it rotates about this central (longitudinal) axis, the moment of inertia is
I = MR2
Example
A hollow cylindrical shell (thin-walled) has:
- Mass M = 6 kg
- Radius R = 0.5 m
For a thin-walled hollow cylinder, all the mass is assumed to be at a fixed distance R from the axis. The moment of inertia is given by:
I = MR2
I = 6 × (0.5)2
I = 6 × 0.25 = 1.5 kg⋅m2
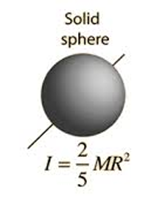
Solid Sphere
A solid sphere is a perfectly round object with mass distributed uniformly throughout its volume. When it rotates about an axis through its centre (like a spinning ball), its moment of inertia is
I = ⅖ MR2
Example
A solid sphere has:
- Mass M = 4 kg
- Radius R = 0.2 m
For a solid sphere rotating about its center (through its diameter), the moment of inertia is:
I = ⅖ MR2
I = ⅖ × 4 × (0.2)2
I = ⅖ × 4 × 0.04
I = 0.064kg⋅m2
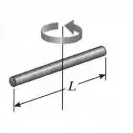
Thin Rod, with axis at the centre
A thin, uniform rod has mass distributed evenly along its length. When it rotates about an axis perpendicular to its length and passing through its centre (like a baton spinning in the air), the moment of inertia is
I = 1/12 ML2
Example
A thin, uniform rod has:
- Mass M = 2 kg
- Length L = 1.5 m
When the rotation axis is at the center of the rod and perpendicular to its length, the moment of inertia is:
I = 1/12 ML2
I = 1/12 × 2 × (1.5)2
I = 1/12 × 2 × 2.25
I = 0.375kg⋅m2
Thin Rod with axis at the end
A thin, uniform rod with rotation about an axis perpendicular to its length and passing through one end (like a door swinging on a hinge) has a moment of inertia given by:
I = ⅓ ML2
Example
A thin rod of length 2 m and mass 3 kg is rotating about one of its ends perpendicular to its length. What is the moment of inertia?
I = ⅓ ML2
Where:
- M 3 kg
- L = 2 m
I = ⅓ × 3 × (2)2
I = 1×4 = 4 kg⋅m2
So, the moment of inertia is 4 kg·m².
The larger the moment of inertia, the harder it is to rotate the object.
Units of moment of inertia: kg⋅m2
Interested in our engineering courses?
We have over 70 courses across all major engineering disciplines, including, mechanical, electrical and electronic, civil, aerospace, industrial, computer and general engineering. Visit our course catalogue for a complete list of fully accredited engineering programmes.
A small selection of short courses …
Diploma in Mechanical Engineering
Diploma in Structural Engineering
Level 6 Courses
International Graduate Diploma in Mechanical Engineering
International Graduate Diploma in Civil Engineering
International Graduate Diploma in Aerospace Engineering
Level 5 Courses
Higher International Diploma in Mechanical Engineering
Higher International Diploma in Civil Engineering
Higher International Diploma in Aerospace Engineering
Level 4 Courses
Higher International Certificate in Mechanical Engineering
Higher International Certificate in Civil Engineering
Higher International Certificate in Aerospace Engineering
Alternatively, you can view all our online engineering courses here.
Recent Posts
Starting Your Engineering Journey: The Higher International Certificate (Level 4)
Starting Your Engineering Journey: The Higher International Certificate (Level 4) Embarking on a career in engineering is a significant decision, and choosing the right starting point can make all the difference. The Higher International Certificate (HIC) from iLearn Engineering® offers a flexible, accessible, and globally recognised route into the profession. Whether you are beginning your […]
Understanding Qualification Levels and Credits: a guide for engineering students
Understanding Qualification Levels and Credits: a guide for engineering students 1. Introduction to Qualification Levels 2. What Are Credits and Why Do They Matter? While 120 credits is broadly equivalent to one academic year, in traditional university settings this would usually be delivered across approximately 39 weeks with fixed timetables. In contrast, asynchronous learning models—such […]
What is Inertia and how to Calculate it ?
What is Inertia and how to Calculate it ? Inertia is the property of matter that causes it to resist changes in its velocity. This includes changes to the object’s speed or direction of motion. Inertia is directly related to mass, the greater the mass, the greater the inertia. In simple terms: “An object in […]

